todayilearned

This paper says nothing about the "brain's navigation system". It is focused on the distribution of cone photoreceptors in the retina, and more precisely in the fovea.

They are indeed organized in a hexagonal mosaic as you can see in the picture, and the authors present a new method to estimate spatial distribution of said cones, showing that there are anisotropies, with the cones having a larger local spacing along the horizontal axis.

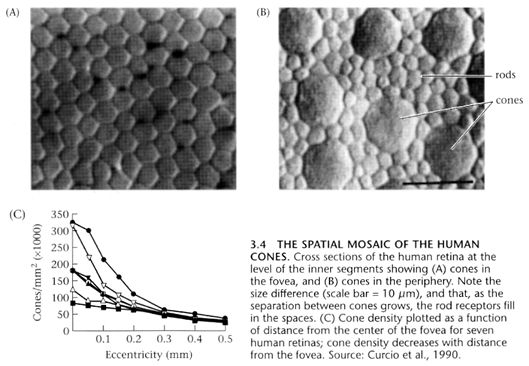
The fovea (A) is the central-ish region of the retina, and it is packed with cones, which are specialized in color recognition. As you move to the periphery of the retina (B), another type of receptor becomes dominant : the rods. They are a lot better at sensing light but can't tell which color it is. That's why our peripheral vision is mostly shades of gray, even if your brain tries to convince you otherwise by adding the colors from context.

Anyway, not saying this isn't an interesting topic, but it's not the best source to illustrate it.

Hexagon are bestagon

Find a more efficient packing pattern.

It makes sense because the real world is isotopic. Rectangular is presumptuous to some extent where high frequency information will be found.