this post was submitted on 16 Nov 2024
384 points (97.5% liked)
Memes
8404 readers
587 users here now
Post memes here.
A meme is an idea, behavior, or style that spreads by means of imitation from person to person within a culture and often carries symbolic meaning representing a particular phenomenon or theme.
An Internet meme or meme, is a cultural item that is spread via the Internet, often through social media platforms. The name is by the concept of memes proposed by Richard Dawkins in 1972. Internet memes can take various forms, such as images, videos, GIFs, and various other viral sensations.
- Wait at least 2 months before reposting
- No explicitly political content (about political figures, political events, elections and so on), !politicalmemes@lemmy.ca can be better place for that
- Use NSFW marking accordingly
Laittakaa meemejä tänne.
- Odota ainakin 2 kuukautta ennen meemin postaamista uudelleen
- Ei selkeän poliittista sisältöä (poliitikoista, poliittisista tapahtumista, vaaleista jne) parempi paikka esim. !politicalmemes@lemmy.ca
- Merkitse K18-sisältö tarpeen mukaan
founded 2 years ago
MODERATORS
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments

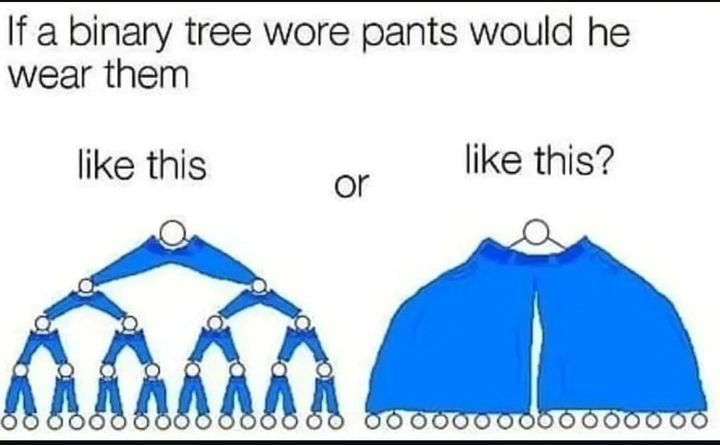
Legit question: which requires the less amount of fabric?
Modeling a single pair of pants
For the sake of the calculation, lets model the pants as a prism, where:
Total fabric for a given pair of pants p would then be A_p = A_tp * 2 + U_p * d
Nomenclature
Lets denote the two ways of wearing the pants as:
First comparison
We can already note that the amount of fabric that is used, for the two possible ways to wear the pants, will depend on how densely the branches of the tree are packed. Assuming a maximally densely packed tree (if such is possible), where there is no space in between any branches, we'd get a the same area for A_t on the single pants for W_1 and the sum of all A_tp over all little pants in W_c:
A_tW1 = sum( A_tp ) for all pants p in W_c
It's clear then, that the area difference between W_1 and W_c then only depends on the circumference U_1 in W_1 VS the circumferences U_p in W_c. In this case, the sum of all U_p for each pair of pants p in W_c must be higher than U_1 in W_1, as long as the binary tree has a depth of at least two. This is because the pants would have to have to cover an exterior outline of at least U_1 and in addition there would interior borders between the pants.
Therefore, for a maximally packed tree, W_1 would use less fabric than W_c. On the other hand, given a tree where the siblings would be far away from each other, there would be a lot of space between the siblings. This would then diminish the areas A_tp for the pants in W_c in comparison to A_1 in W_1. (I know this isn't very rigorous here, but I don't want to go into too much depth here)
From this, we learn that the comparison of W_1 and W_c depends on the packing density of the pants.
Packing density
The packing density depends on the difference in branch length between children and parents within the tree. It also depends on the angle of the bottom branches to each other. For higher nodes, I'd assume that a node will always be horizontally aligned in the middle between it's child nodes. Therefore, the angle is only dependent on the length of the legs for non-bottom nodes.
I need to stop now as I'm hungry
Maybe I'll pick up where I left later.